中科大-凸优化 笔记（lec4）-凸集和凸锥

全部笔记的汇总贴（视频也有传送门）：中科大-凸优化

一、凸集（Convex Set）

仿射集一定是凸集

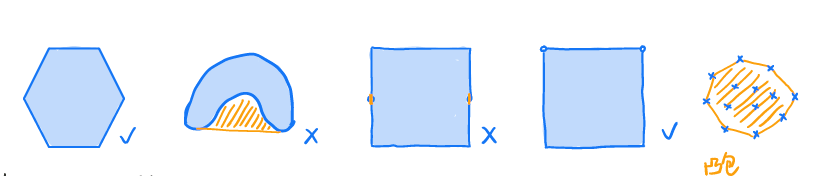
凸集（Convex Set）：一个集合$C$是凸集，当任意两点之间的线段仍在$C$内。

$C$为凸集$\iff$$\forall x_1,x_2\in C,\forall \theta ,\theta \in [0,1],\theta x_1+(1-\theta )x_2\in C$

凸组合：${\theta }_1{x}_1+\cdots +{\theta }_k{x}_k，{\theta }_1,\cdots {\theta }_k\in \mathbb{R}，{\theta }_1+\cdots +{\theta }_k=1，{\theta }_1,\cdots ,{\theta }_k\in [0,1]$

$C$为凸集$\iff$任意元素凸组合$\in C$

凸包：$C\in {\mathbb{R}}^n$
C o n v      C = { θ 1 x 1 + ⋯ + θ k x k ∣ ∀ x 1 , ⋯   , x k ∈ C , ∀ θ 1 , ⋯   , θ k ∈ [ 0 , 1 ] , θ 1 + ⋯ + θ k = 1 } Conv\;\; C=\{\theta_1 x_1+\cdots+\theta_k x_k|\forall x_1,\cdots,x_k\in C,\forall\theta_1,\cdots,\theta_k\in[0,1] ,\theta_1+\cdots+\theta_k=1\} ConvC={ θ1​x1​+⋯+θk​xk​∣∀x1​,⋯,xk​∈C,∀θ1​,⋯,θk​∈[0,1],θ1​+⋯+θk​=1}

二、凸锥（Convex Cone）

$C$是锥$\iff \forall x\in C,\theta \ge 0,$有$\theta x\in C$
$C$是凸锥$\iff \forall x_1,x_2\in C,{\theta }_1,{\theta }_2\ge 0,$有${\theta }_1{x}_1+{\theta }_2{x}_2\in C$

凸锥组合：${\theta }_1{x}_1+\cdots +{\theta }_k{x}_k{\theta }_1,\cdots ,{\theta }_k\ge 0$
凸锥包： x 1 , ⋯   , x k ∈ C      { θ 1 x 1 + ⋯ + θ k x k ∣ x 1 , ⋯   , x k ∈ C , θ 1 , ⋯   , θ k ≥ 0 } x_1,\cdots,x_k\in C\;\;\{\theta_1x_1+\cdots+\theta_kx_k|x_1,\cdots,x_k\in C,\theta_1,\cdots,\theta_k\ge0\} x1​,⋯,xk​∈C{ θ1​x1​+⋯+θk​xk​∣x1​,⋯,xk​∈C,θ1​,⋯,θk​≥0}

仿射组合$\forall {\theta }_1,\cdots ,{\theta }_k{\theta }_1+\cdots +{\theta }_k=1$
凸组合$\forall {\theta }_1,\cdots ,{\theta }_k{\theta }_1+\cdots +{\theta }_k=1,{\theta }_1,\cdots ,{\theta }_k\in [0,1]$
凸锥组合$\forall {\theta }_1,\cdots ,{\theta }_k{\theta }_1+\cdots +{\theta }_k\ge 0$

C = { x }                    θ 1 x + θ 2 x = x                    仿 射 集                  ∅ 也 是 仿 射 集 C=\{x\}\;\;\;\;\;\;\;\;\;\theta_1x+\theta_2x=x\;\;\;\;\;\;\;\;\;仿射集\;\;\;\;\;\;\;\;\varnothing也是仿射集 C={ x}θ1​x+θ2​x=x仿射集∅也是仿射集

$\varnothing$是仿射集、凸集、凸锥。

下一章传送门：中科大-凸优化 笔记（lec5）-几种重要的凸集（上）